• The molecule studied consists of one or more spin subsystems. Within a spin system, the spins interact with the external magnetic fields and with each other. These NMR interactions can be either classically modelled or more generally quantum modelled by associating them with a Hamiltonian.
  
• The Hamiltonian associated with each interaction can be separated into two contributions, one isotropic, the other anisotropic. This latter contribution is by definition zero on average in an isotropic environment, because of the Brownian movement
  

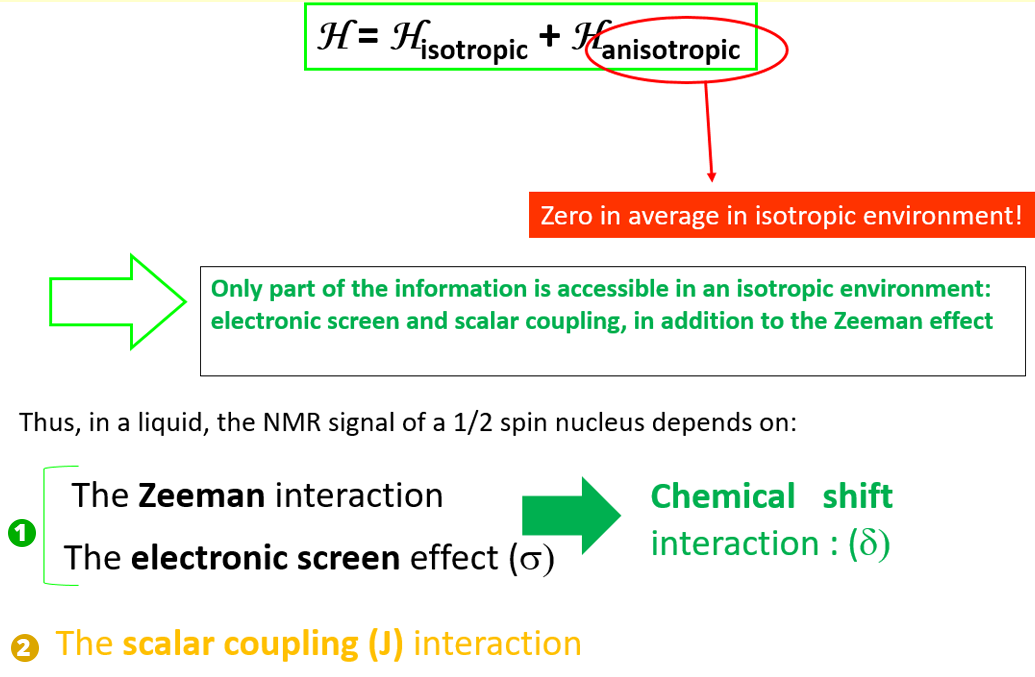
• The anisotropic parts of the chemical shift and the scalar coupling are averaged at zero
• The dipole interaction is instantaneous: it can be exploited even if one does not see its effect through a structure on the spectrum!
• The final Hamiltonian is written:

When the non-secular term has a negligible contribution in relation to secular terms, the spin system is of the first order: the multiplicity rules of Pascal's binomial apply.